|
An interesting property of optimal growth models is the turnpike property, borrowed from multi-sectoral growth models and identified for the Ramsey and Cass-Koopmans models by Paul Samuelson (1965) and David Cass (1966). Suppose that, instead of maximizing an infinite-horizon problem, the social planner considers a model with a finite horizon. In other words, suppose the social welfare function is in fact S = ò 0T u[c(t)]e-(r -n)t dt, where T is the final time period. We can leave the end-point free, or we can in addition impose that, in the final time period, the capital-labor ratio must be at least above a pre-specified level kT. Thus, the social planner's problem becomes:
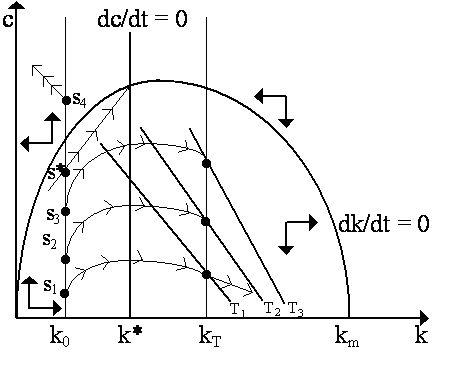
It is evident that the underlying dynamics will be identical, except that now have this pesky terminal point to worry about. The problem can be visualized more or less as in Figure 4. Our initial and minimum final capital-labor ratios are indicated by k0 and kT respectively. The choice our social planner makes is between the various paths that will take us from k0 to kT. The social planner could, for instance, choose initial starting point s1 and ride the disequilibrium path until he reaches kT. Or he choose alternative initial starting points s2 or s3 and ride their corresponding disequilibrium paths to kT. He will not choose initial starting point s4, because it goes in the opposite direction and will not get him to kT at all.
Which is the best path to get to kT? This depends on the amount of time T that is available. To see why, compare the paths defined by s1 and s2: starting at s1, we consume very little, which means that capital accumulation is very quick. In contrast, starting at s2, we consume relatively more, which means that capital accumulation is slower. In terms of utility, s2 should be better than s1 as it yields more consumption over the path. However, if the finite time limit T is very, very short, following the slower path s2 may not take us to our destination kT within the ascribed time. In Figure 4, we draw out several isochronic lines. The facet T1 denotes the combinations of c and k which can be reached at time T1, i.e. where a path crosses T1, that is where we are at that time T1. These lines are downward sloping because different paths have different speeds of capital accumulation. So, in Figure 4, notice that, at time T1, s1 is already at its final destination kT, but the slower paths s2 and s3 are still behind at capital-labor ratios below kT. For every time period, we can define an isochronic line. Thus, line T2 represents the (c, k) combinations that can be reached at T2. Notice that at T2, s2 hits final destination kT, but s3 is still behind while s1 has already overshot it. Thus, in terms of time, T1 < T2 < T3. The story in Figure 4 can be told by depicting the paths explicitly over time as in Figure 5. That paths s1, .., s4 depicted in Figure 5 correspond (roughly) to those in Figure 4. Notice that if we impose time limit T1, then of the depicted paths, only s1 reaches the destination kT, all others are below it. If the time limit is increased to T2, then s1 overshoots, s2 hits kT, while s3 is still far behind. If we increase time to T3, then s3 hits it at last, with s1 and s2 having long overshot it.
So what is the optimal path? This depends on the time horizon. Suppose we impose T2 as the time limit to reach kT. Obviously, overshooting is wasteful: it implies that we have accumulated more capital than necessary within the allotted time, and thereby depressed consumption in the interim more than was necessary. So, s1 is not the optimal path if the time limit is T2. Similarly, s3 does not even reach kT at T2, so it cannot even be considered. So the optimal path is s2 which just hits kT it at time T2, and thus fulfills our endpoint condition and wastes no consumption. Similarly, if the finite time limit is T1, then s1 is our optimal path if the final time limit is T3, then s3 is our optimal path. Suppose, now, for the sake of argument, that there is no time limit at all, so we have an infinite-horizon problem. As we know from Cass-Koopmans, the optimal path will be to jump on the stable arm and go to the steady-state equilibrium k*. This is depicted in Figure 5 by the path s*. Now, one thing that is immediately evident from Figure 5 is that the longer the final time period, the "flatter" the path becomes. Or, alternatively stated, the further away T is, the more the finite-horizon optimal path, call it sT, resembles the optimal infinite-horizon path s*. This is the heart of the turnpike property of optimal growth models. According to the "turnpike" theorem, the optimal path for a finite horizon problem with specified endpoint will be precisely the path that is "closest" to the infinite-horizon path. Thus, of the three paths depicted in Figure 5, the considerably flat s3 "resembles" s* more than the alternative steeper paths s2 or s1. The rest of the turnpike theorem is then that as T ® ¥ , then sT ® s*. The reason for the name of the theorem should be explained. A turnpike is a fast road, and it rarely takes us from exactly where we are to exactly where we want to go. But if we want to get from A to B, then the best route will be to "get on" the turnpike as soon as possible after we leave A, and "get off" it when we are near our destination B, rather than try a slower country road that may connect A and B more directly. In short, to benefit from the superior speed and quality of the turnpike, we might be willing to go out of our way a little bit. The infinite-horizon optimal path, s*, is the analogue to the "turnpike" in the story. Even though s1 is a "more direct" path to meet kT by T3, s3 is nonetheless a much better path. For any given T, of all the feasible finite-horizon paths, the optimal one, sT, is the one that is "closest" to the optimal s* path. Thus, in turnpike behavior, the optimal path is not really to get "on" the turnpike, but rather to "arch" towards it. Models for which this is true are said to have the turnpike property. As we have seen, albeit only intuitively, the Cass-Koopmans optimal growth model is certainly one of them.
|
All rights reserved, Gonšalo L. Fonseca